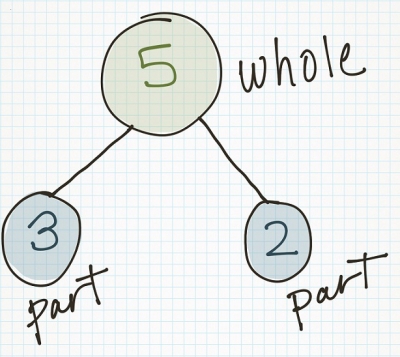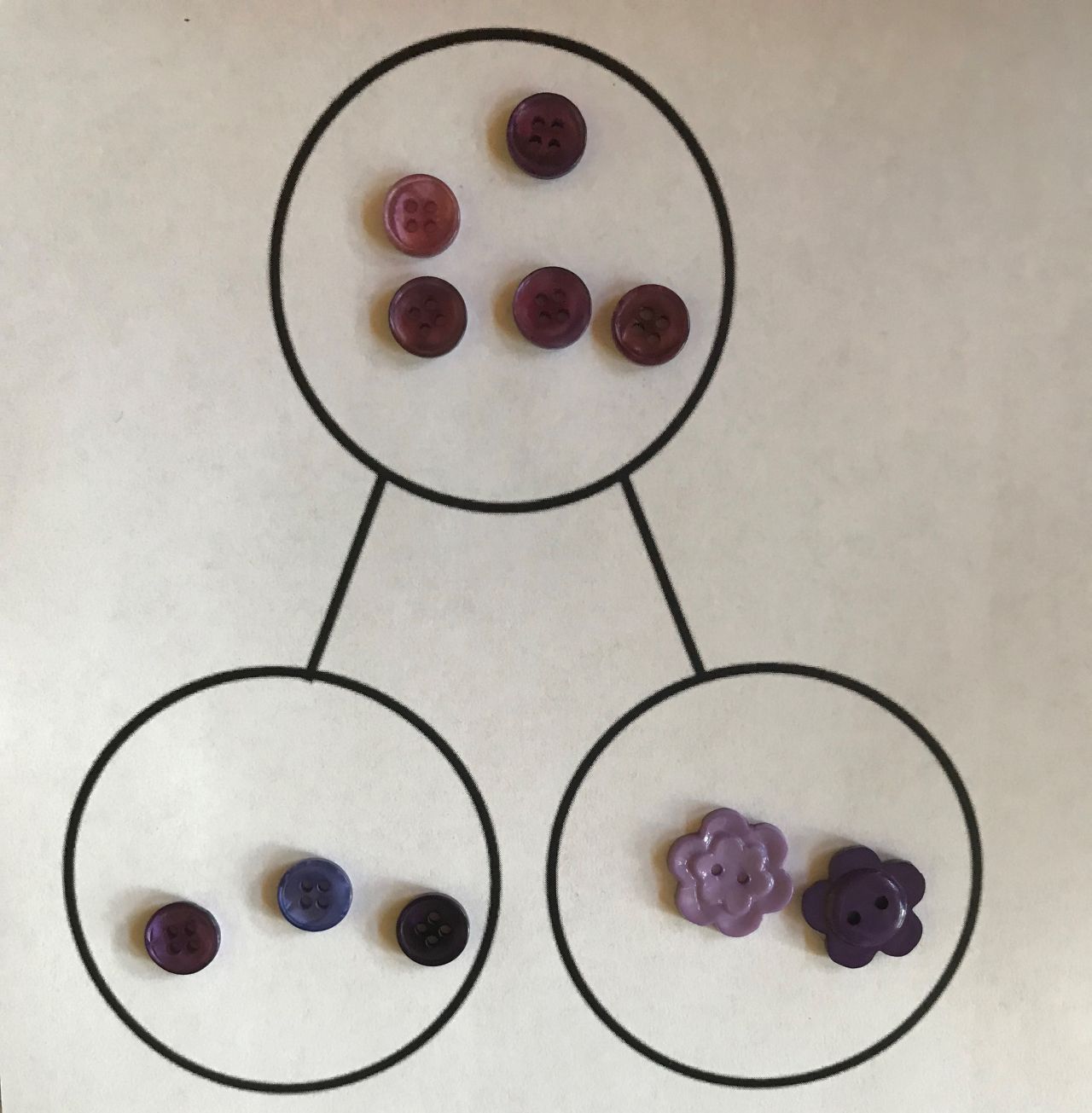
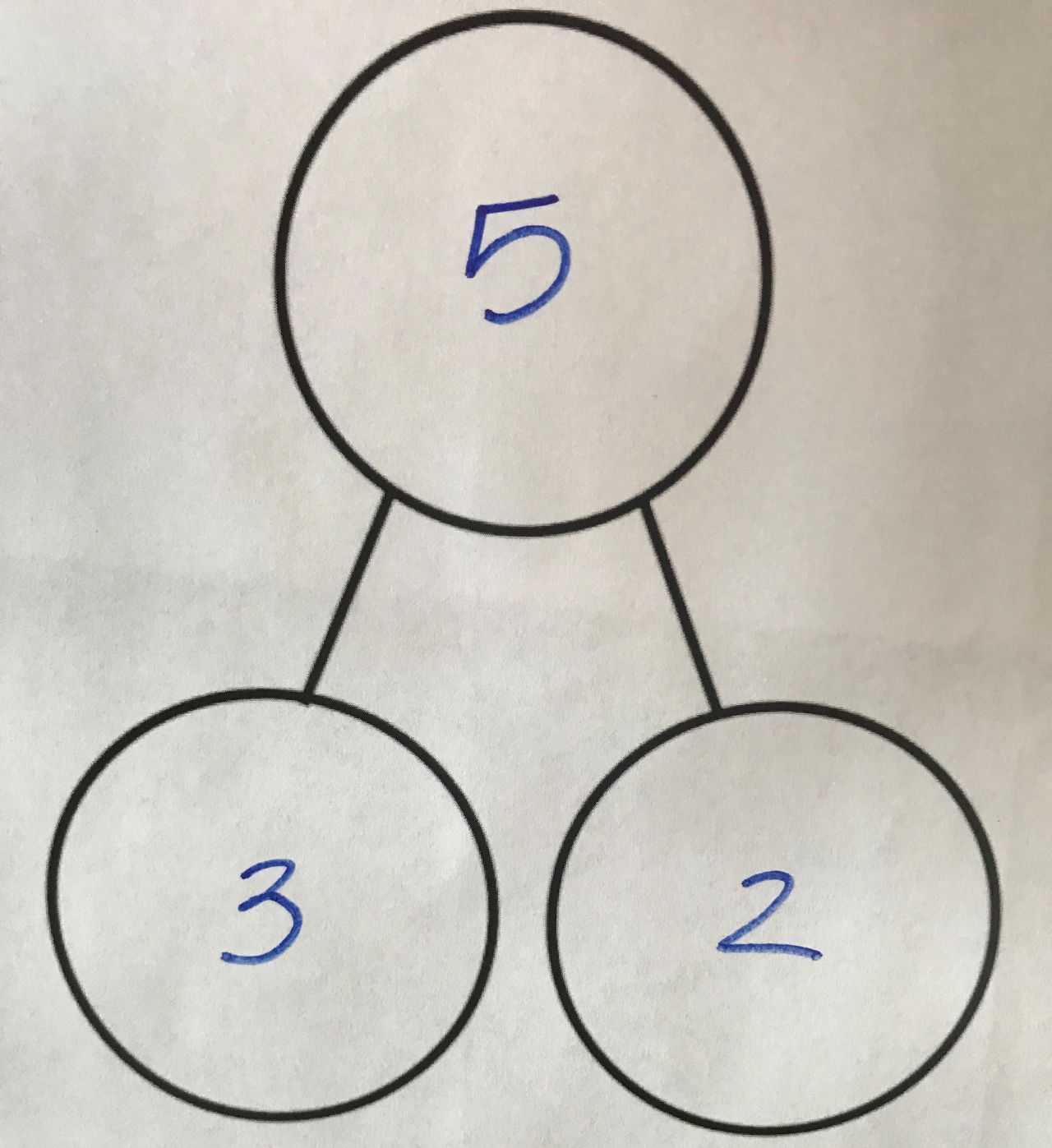
Number bonds in PK-K
Students begin in preschool and kindergarten working with the numbers 1 through 5. Again, while it may seem trivial, it is critically important that a young student understands all the decompositions of each of these whole numbers. Tell me about 5: 5 is 1 and 4. 5 is 2 and 3. 5 is 5 and 0, and so on. Not only does this develop number sense for these important early numbers, it also prepares students to do mental math and understand how this property applies to all numbers.
Number bonds in Grade 1
Late kindergarten and grade 1 are the time when children use number bonds to learn an immensely important group of numbers, the 10 combinations. For students to succeed going forward, they must learn deeply and with fluency and automaticity all the combinations for the decompositions of 10. Again, this may seem easy enough, but time must be taken first to work with concrete objects to construct understanding. Working next with number bonds will serve to internalize these relationships and develop mental math abilities.
Number bonds in Grade 2 and beyond
It is critical that students entering second grade have gained mental math skills of composing and decomposing numbers within 10. In our base 10 number system, we are constantly manipulating the number 10 (or 100, or 1000, or 0.01). This ability begins, as do so many other number skills, in these early years. Hopefully, the use of number bonds does not end in here. This strategy continues to be very powerful as the content progresses.
Singapore mental math: addition in Grade 1
Let's look at the addition strategy of the teen numbers. This can be a hurdle for many students, but thinking in terms of number bonds can help tremendously. Let’s look at 7 + 8, one of the more difficult early addition facts. We see several methods for solving this, including the often-used “counting on,” often involving fingers. This method is not only inefficient, it has a high potential for inaccuracy. What we would rather see is the use of number bonds to find the sum. The mental math process involves finding the number needed to make a 10 with the 7, 3. Then, we decompose the 8 into 3 and 5. 7 and 3 make 10, 10 and 5 make 15. This strategy is sophisticated, accurate, and takes advantages of the properties of base 10.
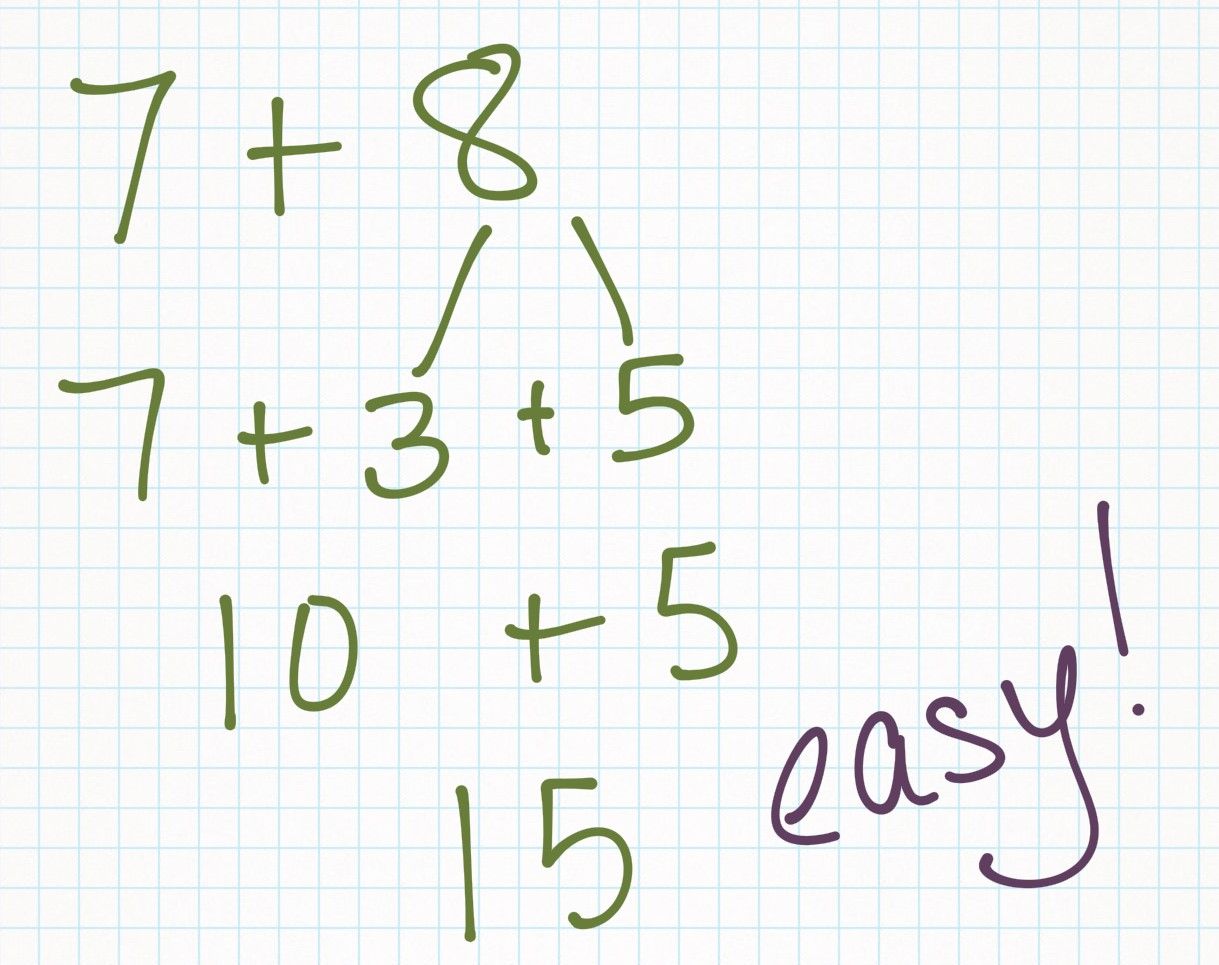
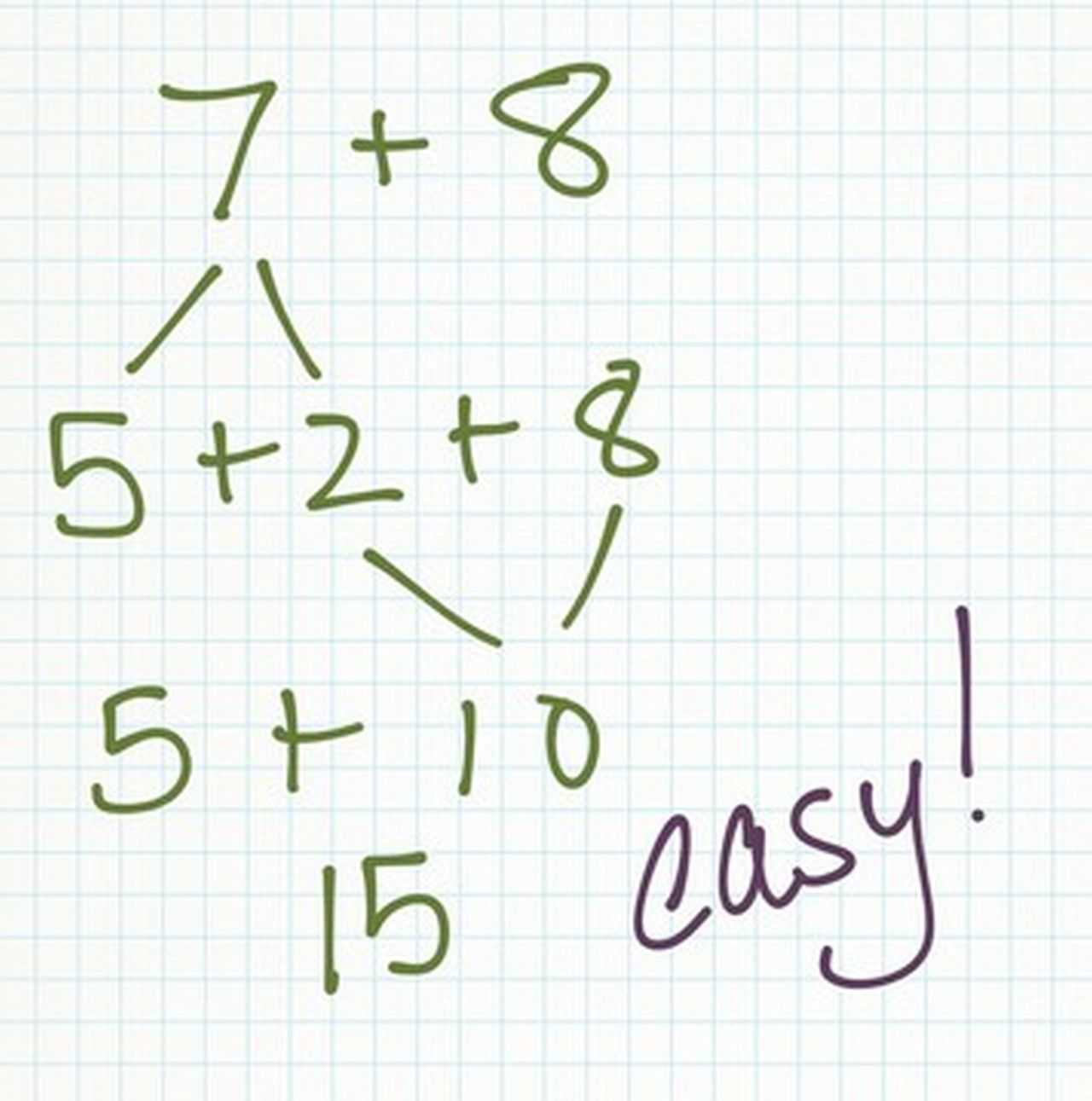
Singapore mental math: subtraction in Grade 1
It works just as well with subtraction. In the land of number bonds, there is no regrouping in the teen numbers. This is one of the most important Singapore mental math strategies that students learn in Grade 1. It involves taking a number away from 10 and then adding the result to the other part. For example, when a student sees 15 – 7, we want them, rather than perform the algorithm, to draw on their understanding of number bonds. Students will take the 7 from the 10, and add the result to the other part. Again, an elegant solution that respects the properties of base 10. It also keeps us out of the endless loop we have all seen so often:
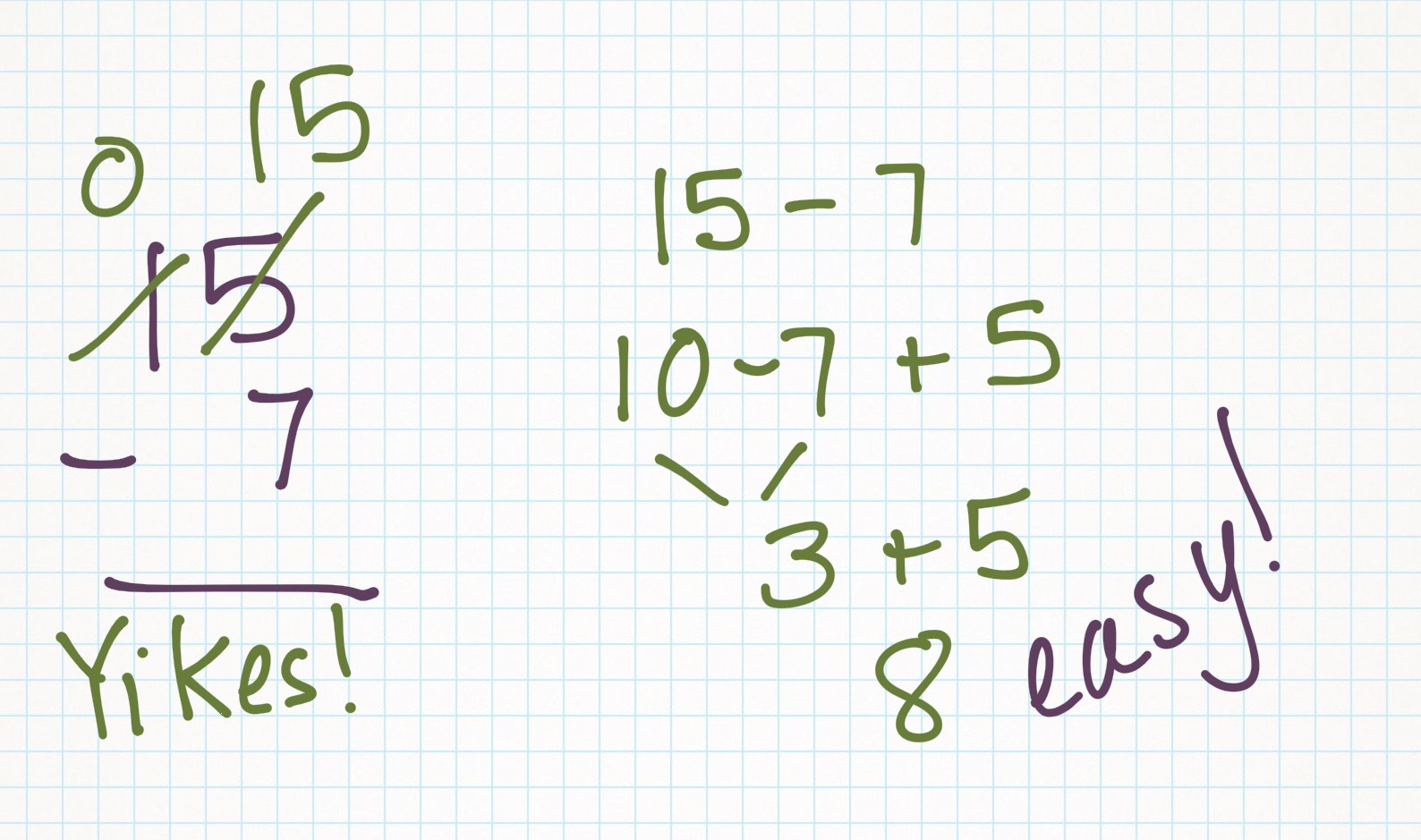
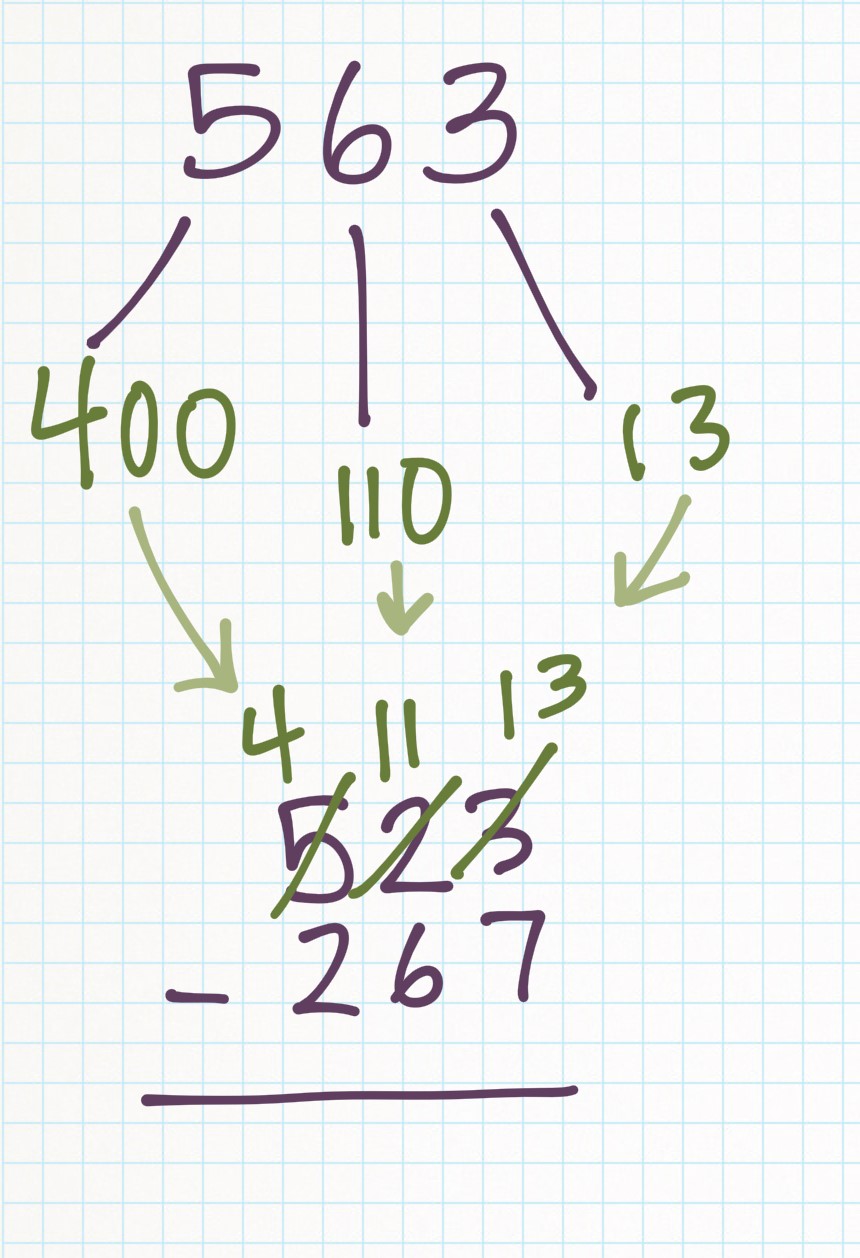
Number bonds can be extremely useful as the number of place values increases, and students learn to use the addition and subtraction algorithms. Just as numbers can be decomposed into more than 2 parts, our number bonds can have 3 or more parts. Consider subtraction with regrouping in several places. Before students can do the algorithm, they need to understand the algorithm. Number bonds can be extremely helpful in this endeavor. For example, when we subtract 267 from 523, we do this:
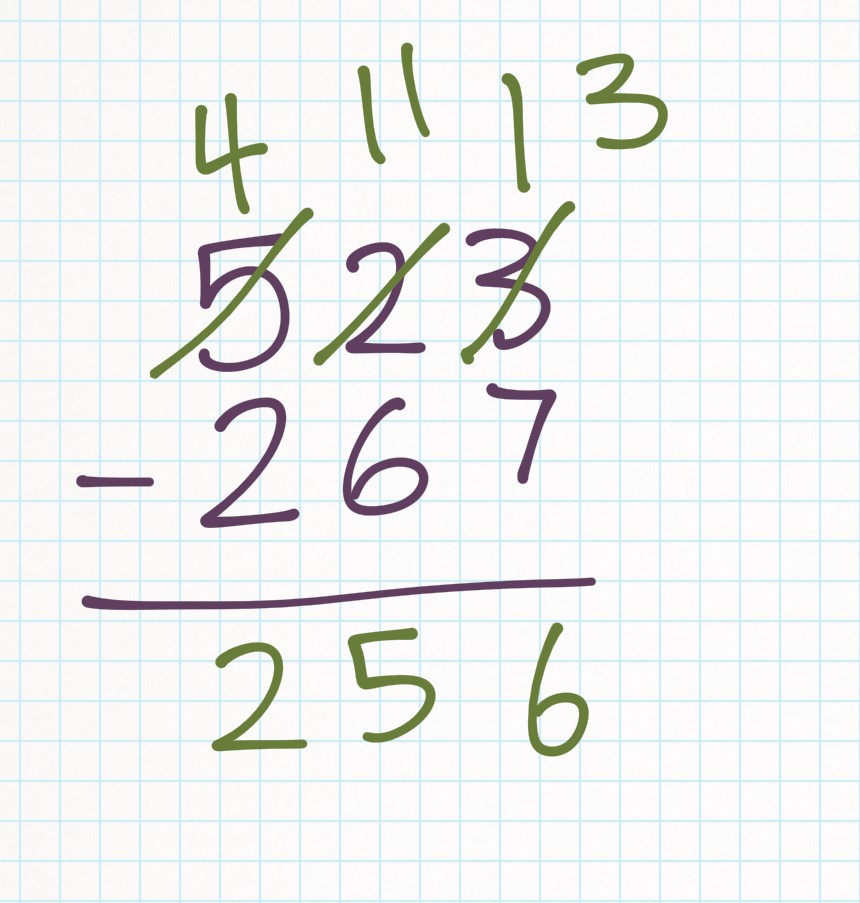
But, what are we really doing? We are decomposing 523 into a number bond that suits our needs. The number bond using the place value will not help us. But, the one where we regroup will get the job done. Finding the connection between these number bonds and those mysterious tiny numbers written in the algorithm will be a tremendous help in understanding the algorithm.
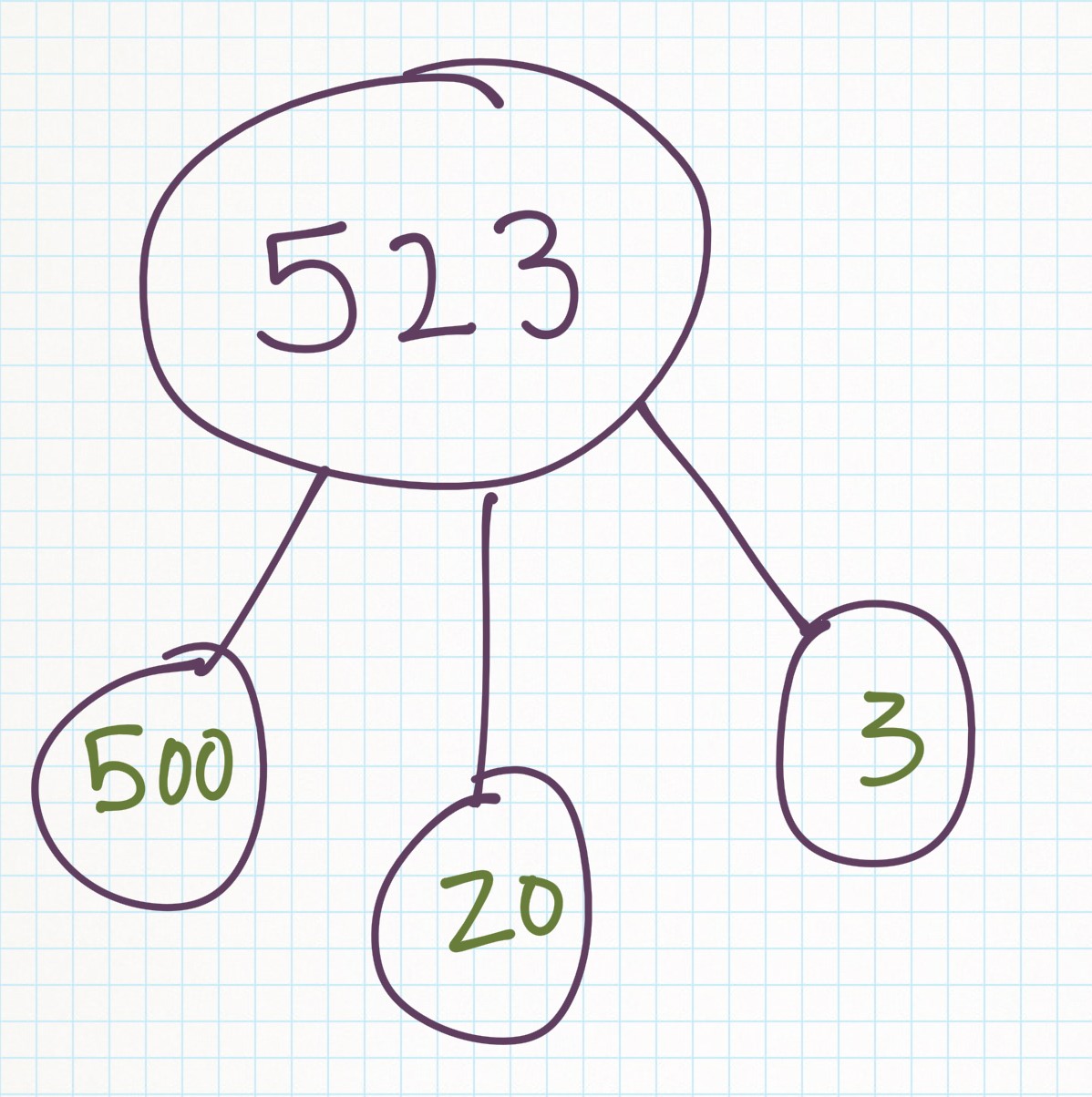
The same goes for addition. When we perform the algorithm, we are actually creating a number bond for the parts that make up the final sum. Like this one:

Looking ahead
Because students have developed and internalized the Singapore Math strategies of decomposing quantities in the context of number bonds, these powerful skills can then be applied to many other contexts in the years to come. Multiplication, division, and fractions are but a few. The importance and value of this skill set can not be overstated. It is well worth the time spent in the early years to establish this important habit of mind about numbers.